Last updated on March 1st, 2025 at 09:41 pm
Here, we see a Cut Off Trees for Golf Event LeetCode Solution. This Leetcode problem is solved using different approaches in many programming languages, such as C++, Java, JavaScript, Python, etc.
List of all LeetCode Solution
Topics
Breadth-First Search
Companies
Amazon
Level of Question
Hard

Cut Off Trees for Golf Event LeetCode Solution
Table of Contents
1. Problem Statement
You are asked to cut off all the trees in a forest for a golf event. The forest is represented as an m x n matrix. In this matrix:
0means the cell cannot be walked through.1represents an empty cell that can be walked through.- A number greater than
1represents a tree in a cell that can be walked through, and this number is the tree’s height.
In one step, you can walk in any of the four directions: north, east, south, and west. If you are standing in a cell with a tree, you can choose whether to cut it off.
You must cut off the trees in order from shortest to tallest. When you cut off a tree, the value at its cell becomes 1 (an empty cell).
Starting from the point (0, 0), return the minimum steps you need to walk to cut off all the trees. If you cannot cut off all the trees, return -1.
Note: The input is generated such that no two trees have the same height, and there is at least one tree needs to be cut off.
Example 1:

Input: forest = [[1,2,3],[0,0,4],[7,6,5]]
Output: 6
Explanation: Following the path above allows you to cut off the trees from shortest to tallest in 6 steps.
Example 2:
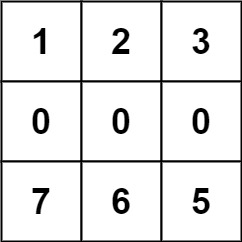
Input: forest = [[1,2,3],[0,0,0],[7,6,5]]
Output: -1
Explanation: The trees in the bottom row cannot be accessed as the middle row is blocked.
Example 3:
Input: forest = [[2,3,4],[0,0,5],[8,7,6]]
Output: 6
Explanation: You can follow the same path as Example 1 to cut off all the trees. Note that you can cut off the first tree at (0, 0) before making any steps.
2. Coding Pattern Used in Solution
The coding pattern used in all the provided implementations is Tree Breadth-First Search (BFS). The problem involves navigating a grid (treated as a 2D matrix) to find the shortest path between points, which is a classic BFS problem. BFS is used to calculate the minimum distance between the current position and the target tree in the forest.
3. Code Implementation in Different Languages
3.1 Cut Off Trees for Golf Event C++
class Solution {
static constexpr int DIR[4][2] = {{-1,0},{1,0},{0,-1},{0,1}};
struct Cell {
short r : 8;
short c : 8;
};
int doit(const vector<vector<int>>& forest, Cell start, vector<int> &curr, vector<int> &prev, vector<Cell> &bfs) {
const int M = forest.size(), N = forest[0].size();
int steps = 0;
swap(curr, prev);
fill(begin(curr), end(curr), -1);
curr[start.r * N + start.c] = steps;
if (prev[start.r * N + start.c] != -1) {
return prev[start.r * N + start.c];
}
bfs.clear();
bfs.push_back(start);
while (!bfs.empty()) {
int size = bfs.size();
steps++;
while (size--) {
auto [r0, c0] = bfs[size];
swap(bfs[size], bfs.back());
bfs.pop_back();
for (auto [dr, dc] : DIR) {
short r1 = r0 + dr, c1 = c0 + dc;
int pos = r1 * N + c1;
if (r1 >= 0 && r1 < M && c1 >= 0 && c1 < N && forest[r1][c1] > 0 && curr[pos] == -1) {
if (prev[pos] != -1) {
return steps + prev[pos];
}
curr[pos] = steps;
bfs.push_back({r1, c1});
}
}
}
}
return -1;
}
int manhattan_distance(vector<Cell> &cells) {
int result = 0;
Cell prev{0, 0};
for (auto &cell : cells) {
result += abs(prev.r - cell.r) + abs(prev.c - cell.c);
prev = cell;
}
return result;
}
public:
int cutOffTree(vector<vector<int>>& forest) {
const int M = forest.size(), N = forest[0].size();
if (forest[0][0] == 0) {
return -1;
}
int obstacles = 0;
vector<Cell> cells;
cells.reserve(8);
for (short r = 0; r < M; r++) {
for (short c = 0; c < N; c++) {
if (forest[r][c] > 1) {
cells.push_back({r, c});
} else if (forest[r][c] == 0) {
obstacles++;
}
}
}
sort(begin(cells), end(cells), [&forest](const Cell &a, const Cell &b){
return forest[a.r][a.c] < forest[b.r][b.c];
});
if (obstacles == 0) {
return manhattan_distance(cells);
}
vector<int> curr(M * N, -1), prev = curr;
curr[0] = 0;
vector<Cell> bfs;
bfs.reserve(8);
int steps = 0;
for (auto &cell : cells) {
int result = doit(forest, cell, curr, prev, bfs);
if (result != -1) {
steps += result;
} else {
return -1;
}
}
return steps;
}
};
3.2 Cut Off Trees for Golf Event Java
class Solution {
public int cutOffTree(List<List<Integer>> forest) {
PriorityQueue<int[]> pq=new PriorityQueue<>((a,b)->(forest.get(a[0]).get(a[1])-forest.get(b[0]).get(b[1])));
for(int i=0;i<forest.size();i++){
for(int j=0;j<forest.get(0).size();j++){
if(forest.get(i).get(j)>1)
pq.add(new int[]{i,j});
}
}
int ans=0;
int curr[]={0,0};
while(pq.size()>0){
int[] temp=pq.poll();
int dis=calcDis(forest,curr,temp);
if(dis==-1)
return -1;
ans+=dis;
curr=temp;
}
return ans;
}
int calcDis(List<List<Integer>> forest,int start[],int end[]){
int n=forest.size(),m=forest.get(0).size();
boolean vis[][]=new boolean[n][m];
Queue<int[]> queue=new LinkedList<>();
queue.add(start);
vis[start[0]][start[1]]=true;
int dis=0;
while(queue.size()>0){
int len =queue.size();
while(len-->0){
int temp[]=queue.remove();
int r=temp[0],c=temp[1];
if(r==end[0] && c==end[1])
return dis;
if(r+1<n && !vis[r+1][c] && forest.get(r+1).get(c)!=0){
queue.add(new int[]{r+1,c});
vis[r+1][c]=true;
}if(r-1>=0 && !vis[r-1][c] && forest.get(r-1).get(c)!=0){
queue.add(new int[]{r-1,c});
vis[r-1][c]=true;
}if(c-1>=0 && !vis[r][c-1] && forest.get(r).get(c-1)!=0){
queue.add(new int[]{r,c-1});
vis[r][c-1]=true;
}if(c+1<m && !vis[r][c+1] && forest.get(r).get(c+1)!=0){
queue.add(new int[]{r,c+1});
vis[r][c+1]=true;
}
}
dis++;
}
return -1;
}
}
3.3 Cut Off Trees for Golf Event JavaScript
var cutOffTree = function (forest) {
let trees = forest.flat().filter(x => x && x !== 1).sort((a, b) => b - a);
let currPos = [0, 0], totalDist = 0;
while (trees.length) {
let grid = [...forest.map(row => [...row])];
let ans = getDist(currPos, trees.pop(), grid);
if (ans == null) return -1;
let [pos, dist] = ans;
currPos = pos;
totalDist += dist;
}
return totalDist;
function getDist(start, target, grid) {
let dir = [[1, 0], [-1, 0], [0, 1], [0, -1]];
let queue = [start], dist = 0;
while (queue.length) {
let next = [];
for (let [r, c] of queue) {
if (grid[r][c] === target) return [[r, c], dist];
if (!grid[r][c]) continue;
for (let [x, y] of dir) {
x += r; y += c;
if (x >= 0 && x < grid.length && y >= 0 &&
y < grid[0].length && grid[x][y]) next.push([x, y])
}
grid[r][c] = 0;
}
dist++;
queue = next;
}
return null;
}
};
3.4 Cut Off Trees for Golf Event Python
class Solution(object):
def cutOffTree(self, forest):
heap = []
for r in range(len(forest)):
for c in range(len(forest[0])):
if forest[r][c] > 1:
heapq.heappush(heap, (forest[r][c], r, c))
totalSteps, currR, currC = 0, 0, 0
while heap:
height, r, c = heapq.heappop(heap)
steps = self.bfsGetMinSteps(forest, currR, currC, r, c)
if steps == -1:
return -1
currR, currC = r, c
totalSteps += steps
return totalSteps
def bfsGetMinSteps(self, forest, currR, currC, targetR, targetC):
directions = [(-1, 0), (1, 0), (0, -1), (0, 1)]
m, n = len(forest), len(forest[0])
q = collections.deque([(currR, currC)])
visited = set([(currR, currC)])
steps = 0
while q:
sameLevelCounts = len(q)
for _ in range(sameLevelCounts):
r, c = q.popleft()
if r == targetR and c == targetC:
return steps
for dr, dc in directions:
nextR, nextC = r + dr, c + dc
if 0 <= nextR < m and 0 <= nextC < n and (nextR, nextC) not in visited and forest[nextR][nextC] != 0:
visited.add((nextR, nextC))
q.append((nextR, nextC))
steps += 1
return -1
4. Time and Space Complexity
| Time Complexity | Space Complexity | |
| C++ | O(T * M * N) | O(M * N) |
| Java | O(T * M * N) | O(M * N) |
| JavaScript | O(T * M * N) | O(M * N) |
| Python | O(T * M * N) | O(M * N) |
where, T is the number of trees and M and N are the dimensions of the grid.